Тригонометрические функции
Тригонометрические функции
Свойства тригонометрических функций
Основные тригонометрические тождества
Формулы приведения
Арккосинус
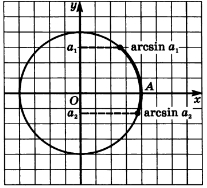
Арксинус
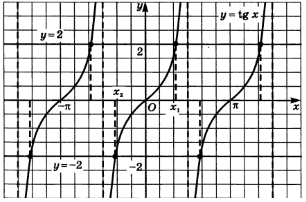
Арктангенс
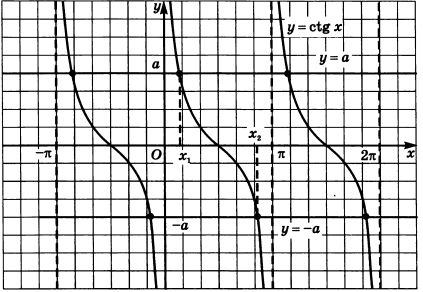
Арккотангенс
Простейшие тригонометрические уравнения
Методы решения тригонометрических уравнений
Однородные тригонометрические уравнения первой степени
Однородные тригонометрические уравнения второй степени
Синус и косинус суммы и разности аргументов
Тангенс суммы и разности аргументов
Формулы двойного аргумента
Производная
Предел функции на бесконечности
Предел функции в точке
Приращение функции
Производная
Формулы дифференцирования
Правила дифференцирования
Уравнение касательной к графику функции
Исследование функций на монотонность
Точки экстремума функции
Нахождение наибольшего и наименьшего значений
Тест по теме: Формулы двойного аргумента
Теория:
Тестовые вопросы и практика:
Напишите формулу
Докажите
Докажите
Напишите формулу
Докажите
пример
Упростите выражение
пример
пример
Упростите выражение
пример
Упростите выражение
Упростите
пример
Упростите
Упростите
\cos 30^{\circ}{n}
Упростите
\sin 30^{\circ}{n}\sqrt{2}{n}